Checked content

File:TaylorCosCos.png

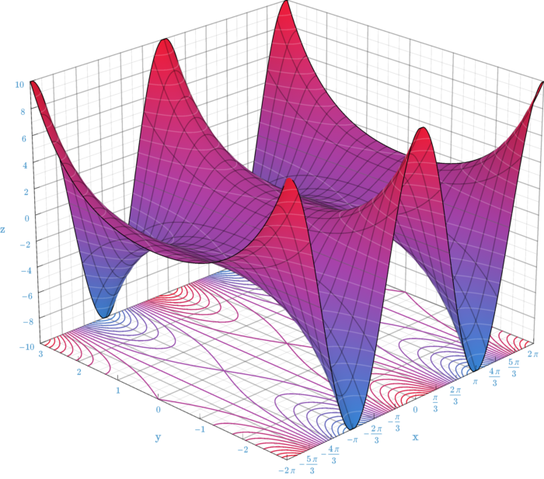
Description Graph of z = Re(cos(x+iy)), selfmade with MuPad
Date 2006-11-25 (original upload date)
Source Originally from en.wikipedia; description page is/was here.
Author Original uploader was Sam Derbyshire at en.wikipedia
Permission
( Reusing this file)

Released under the GNU Free Documentation License.

Licensing

GNU head Permission is granted to copy, distribute and/or modify this document under the terms of the GNU Free Documentation License, Version 1.2 or any later version published by the Free Software Foundation; with no Invariant Sections, no Front-Cover Texts, and no Back-Cover Texts. A copy of the license is included in the section entitled GNU Free Documentation License.

w:en:Creative Commons
attribution share alike		 This file is licensed under the Creative Commons Attribution-Share Alike 3.0 Unported license.
You are free:
  • to share – to copy, distribute and transmit the work
  • to remix – to adapt the work
Under the following conditions:
  • attribution – You must attribute the work in the manner specified by the author or licensor (but not in any way that suggests that they endorse you or your use of the work).
  • share alike – If you alter, transform, or build upon this work, you may distribute the resulting work only under the same or similar license to this one.
This licensing tag was added to this file as part of the GFDL licensing update.
I, the copyright holder of this work, hereby publish it under the following license:
GNU head Permission is granted to copy, distribute and/or modify this document under the terms of the GNU Free Documentation License, Version 1.2 or any later version published by the Free Software Foundation; with no Invariant Sections, no Front-Cover Texts, and no Back-Cover Texts.
Subject to disclaimers.
The following pages on Schools Wikipedia link to this image (list may be incomplete):

Metadata

This file contains additional information, probably added from the digital camera or scanner used to create or digitize it. If the file has been modified from its original state, some details may not fully reflect the modified file.
Software used
  • Wolfram Mathematica 8.0
Date and time of digitizing
  • 02:54, 20 February 2012

What is Schools Wikipedia?

SOS Childrens Villages has brought Wikipedia to the classroom. SOS Children's Villages believes education is an important part of a child's life. That's why we ensure they receive nursery care as well as high-quality primary and secondary education. When they leave school, we support the children in our care as they progress to vocational training or higher education. Sponsoring a child is the coolest way to help.